Interview questions and answer of Clocks with explanation for fresher

Interview questions and answer of Clocks with explanation for fresher

The dial of a clock is a circle whose circumference is divided into 12 parts, called hour spaces. Each hour space is further divided into 5 parts, called minute spaces. This way, the whole circumference is divided into 12 X 5 = 60 minute spaces.

 The time taken by the hour hand (smaller hand) to  cover a distance of an hour space is equal to the time taken by the minute hand (longer hand) to cover a distance of the whole circumference. Thus, we may conclude that in 60 minutes, the minute hand gains 55 minutes on the hour hand.
 Note : The above statement (underlined) is very much useful in solving the problems in this chapter, so it should be remembered. The above statement wants to say that :

 “ In an hour, the hour-hand moves a distance of 5 minute spaces whereas the minute-hand a distance of 60 minute spaces. Thus the minute-hand remains 60 - 5 = 55 minute spaces ahead of the hour-hand.”

Some other facts :

1. In every hour, both the hands coincide once.
2. When the two hands are at right angle, they are 15 minute spaces apart. This happens twice in every hour.
3. When the hands are in opposite directions, they are 30 minute spaces apart. This happens once in every hour.
4. The hands are in the same straight line when they are coincident or opposite to each other.
5. The hour hand moves around the whole circumference of clock once in 12 hours. So the minute hand is twelve times faster than hour hand.
6. The clock is divided into 60 equal minute divisions.
7. 1 minute division = 360060=60${\displaystyle \frac{{360}^0}{60}=6^0}$ apart
8. The clock has 12 hours numbered from 1 to 12 serially arranged.
9. Each hour number evenly and equally separated by five minute divisions 5×60=300$5\times 6^0={30}^0$  apart.
10. In one minute, the minute hand moves one minute division or 60$6^0$.
11. In one minute, the hour hand moves 120${\displaystyle \frac{1}{2^0}}$
12. In one minute the minute hand gains 5120$5{\displaystyle \frac{1}{2^0}}$ more than hour hand.
13. When the hands are together, they are 00$0^0$ apart. Hence,

Too Fast And Too Slow :
 If a watch indicates 9.20, when the correct time is 9.10, it is said to be 10 minutes too fast. And if  is said to be 10 minutes too fast. And if it indicates 9.00, when the correct time is 9.10, it is said to be 10 minutes too slow.

2 important shortcut techniques:

The angle between the hour hand and minute hand at a given time H:MM is given by θ=∣∣∣30×H−112×MM∣∣∣$\theta =\left|30\times H-{\displaystyle \frac{11}{2}\times MM}\right|$

The time after H hours, hour hand and minute hand are at θ$\theta$ degrees = MM=211×(30×H±θ)$MM={\displaystyle \frac{2}{11}\times \left(30\times H\pm \theta \right)}$

Remember any angle less than 180 degrees comes 2 times in 24 hours.

Practice Problems

1. At what time, in minutes, between 3o’ clock and 4o’clock, both the needles will  coincide each other
At 3o’clock, the minute hand is 15 min. spaces apart from the hour hand. To be coincident, it must gain 15 min. spaces.
55 min. are gained in 60 min.
15 min. are gained in (1555×60)$\left({\displaystyle \frac{15}{55}\times 60}\right)$ =16411$=16{\displaystyle \frac{4}{11}}$ min
The hands are coincident at 16411$16{\displaystyle \frac{4}{11}}$  min. past 3.

Alternate method:

We can also solve this problem using degrees.  At 3'O clock, Hour hand and minute hand are seperated by 90 degrees.  Now to meet the Hour hand minute hand has to gain 90 degrees.  We know that for every minute, minute hand gains 5120$5{\displaystyle {\frac{1}{2}}^0}$.  To gain 90 degree it takes 9005120=90112=90×211=18011⇒16411${\displaystyle \frac{{90}^0}{5{{\displaystyle \frac{1}{2}}}^0}={\displaystyle \frac{90}{{\displaystyle \frac{11}{2}}}=90\times {\displaystyle \frac{2}{11}={\displaystyle \frac{180}{11}\Rightarrow 16{\displaystyle \frac{4}{11}}}}}}$ minutes

Alternate method:

Use formula θ$\theta$ degrees = MM=211×(30×H±θ)$MM={\displaystyle \frac{2}{11}\times \left(30\times H\pm \theta \right)}$

MM=211×(30×3±0)=18011=16411$MM={\displaystyle \frac{2}{11}\times \left(30\times 3\pm 0\right)={\displaystyle \frac{180}{11}=16{\displaystyle \frac{4}{11}}}}$ min

2. At what time between 7 and 8o’clock will the hands of a clock be in the same straight line but, not together ?
When the hands of the clock are in the same straight line but not together, they are 30 minute spaces apart.  At 7o’clock, they are 25 min. spaces apart.
 Minute hand will have to gain only 5 min. spaces
55 min. spaces are gained in 60 min.
5 min. spaces are gained in (555×60)$\left({\displaystyle \frac{5}{55}\times 60}\right)$⇒5511$\Rightarrow 5{\displaystyle \frac{5}{11}}$ min. past 7

Alternate method:
At 7'O clock minute hand and hour hand are 150 degrees apart. To be in the same line minute hand has to gain another 30 degrees.  But we know that miute hand gains 5120$5{\displaystyle {\frac{1}{2}}^0}$ degrees per minute.  So 3005120=30112=30×211=6011⇒5511${\displaystyle \frac{{30}^0}{5{{\displaystyle \frac{1}{2}}}^0}={\displaystyle \frac{30}{{\displaystyle \frac{11}{2}}}=30\times {\displaystyle \frac{2}{11}={\displaystyle \frac{60}{11}\Rightarrow 5{\displaystyle \frac{5}{11}}}}}}$

Alternate method:

Use formula θ$\theta$ degrees = MM=211×(30×H±θ)$MM={\displaystyle \frac{2}{11}\times \left(30\times H\pm \theta \right)}$

MM=211×(30×7±180)=210±18011=39011or3011$MM={\displaystyle \frac{2}{11}\times \left(30\times 7\pm 180\right)={\displaystyle \frac{210\pm 180}{11}={\displaystyle \frac{390}{11}or{\displaystyle \frac{30}{11}}}}}$

We regect 39011${\displaystyle \frac{390}{11}}$ as hour hand and minute hand are at 0 degrees.

3. The minute hand of a clock overtakes the hour hand at intervals of 65 minutes of correct time.  How much does the clock gain or lose per day?
If a clock is running on time, Its hour hand and minute hand meets exactly for every 65511$65{\displaystyle \frac{5}{11}}$ min.
But in this clock both hand are meeting at intervals of 65 min. so this clock is gaining time.
or 65 minutes in the correct clock = 65511$65{\displaystyle \frac{5}{11}}$ minutes  in this clock.
Or for every 65 minutes this clock is gaining 5/11 minutes.
for every minute this clock is gaining 511×165${\displaystyle \frac{5}{11}\times \frac{1}{65}}$
In 24 hours or 1440 min it gains = 511×165×24×60${\displaystyle \frac{5}{11}\times \frac{1}{65}\times 24\times 60}$ = 1010143$10{\displaystyle \frac{10}{143}}$

Alternatively:
The minute hand of a clock overtakes the hour hand at intervals of M minutes of correct time. The clock gains or loses in a day by (72011−M)(60×24M)$\left({\displaystyle \frac{720}{11}}-M\right)\left({\displaystyle \frac{60\times 24}{M}}\right)$ minutes.

4. A watch which gains time uniformly is 5 minutes slow at 8'O clock in the morning on Sunday and is 5 minutes 48 seconds fast at 8 PM the following Sunday.  When was it correct?
This sunday morning at 8:00 AM, the watch is 5 min. Slow, and the next sunday at 8:00PM it becomes 5 min 48 sec fast.  The watch gains 5 + 54860$5{\displaystyle \frac{48}{60}}$ = 545${\displaystyle \frac{54}{5}}$ min in a time of  (7×24)+12$\left(7\times 24\right)+12$ = 180 hours.
To show the correct tine, it has to gain 5 min.
545${\displaystyle \frac{54}{5}}$ min  -------180 hours
5 min -------?
⇒5545×180$\Rightarrow {\displaystyle \frac{5}{{\displaystyle \frac{54}{5}}}\times 180}$
⇒8313hrs=72hrs+1113hrs=3days+11hrs+20min$\Rightarrow 83{\displaystyle \frac{1}{3}\mathrm{h}\mathrm{r}\mathrm{s}=72\mathrm{h}\mathrm{r}\mathrm{s}+11{\displaystyle \frac{1}{3}\mathrm{h}\mathrm{r}\mathrm{s}=3\mathrm{d}\mathrm{a}\mathrm{y}\mathrm{s}+11\mathrm{h}\mathrm{r}\mathrm{s}+20\mathrm{m}\mathrm{i}\mathrm{n}}}$

So the correct time will be shown on wednesdy at 7:20 PM

5. A clock is set right at 8 AM.  The clock gains 10 minutes in 24 hours. What will be the true time when the clock indicates 1 PM the following day?
Between 8 AM and 1 PM total 29 hours have passed.
This clock shows 24 hr 10 min or 1456${\displaystyle \frac{145}{6}}$ for 24 hours in correct clock.  In 29 hours in this clock = 291456×24${\displaystyle \frac{29}{{\displaystyle \frac{145}{6}}}\times 24}$ hours in actual clock = 1445${\displaystyle \frac{144}{5}}$ = 2845$28{\displaystyle \frac{4}{5}}$ = 28 hrs 48 min.
So actual time is 12: 48 PM

6. How much does a watch lose per day, if its hands coincide every 64 minutes?
Ans: If a clock is running on time, Its hour hand and minute hand meets exactly for every 65511$65{\displaystyle \frac{5}{11}}$ min.
But in this clock both hand are meeting at intervals of 64 min. So this clock is gaining time.
or 64 minutes in the correct clock = 65511$65{\displaystyle \frac{5}{11}}$ minutes in this clock.
Or for every 64 minutes this clock is gaining 1511$1{\displaystyle \frac{5}{11}}$ minutes. or 1611${\displaystyle \frac{16}{11}}$ minutes
For every minute this clock is gaining 1611×164${\displaystyle \frac{16}{11}}\times {\displaystyle \frac{1}{64}}$
In 24 hours or 1440 min it gains = 1611×164×1440${\displaystyle \frac{16}{11}}\times {\displaystyle \frac{1}{64}}\times 1440$ = 32811$32{\displaystyle \frac{8}{11}}$ minutes

Alternatively:
The minute hand of a clock overtakes the hour hand at intervals of M minutes of correct time. The clock gains or loses in a day by (72011−M)(60×24M)$\left({\displaystyle \frac{720}{11}}-M\right)\left({\displaystyle \frac{60\times 24}{M}}\right)$ minutes.
So ⇒(72011−M)(60×24M)=(72011−64)(60×2464)$\Rightarrow \left({\displaystyle \frac{720}{11}}-M\right)\left({\displaystyle \frac{60\times 24}{M}}\right)=\left({\displaystyle \frac{720}{11}}-64\right)\left({\displaystyle \frac{60\times 24}{64}}\right)$ = 1611(60×38)=211(60×3)${\displaystyle \frac{16}{11}}\left({\displaystyle \frac{60\times 3}{8}}\right)={\displaystyle \frac{2}{11}}\left(60\times 3\right)$ = =36011=32811$={\displaystyle \frac{360}{11}}=32{\displaystyle \frac{8}{11}}$

Level - 2

7. A person who left home between 4 p.m. and 5 p.m. returned between 5 p.m. and 6 p.m. and found that the hands of his watch had exactly changed places. When did he go out?
We know that the dial of the clock has 60 equal divisions (Minute divisions).  In one hour the minute hand makes one complete revolution, i.e., it moves through 60 divisions, and the hour hand moves trough 5 divisions,.  Suppose that when the man went out the hour-hand was x divisions ahead after 4'O clock.  Also suppose that when the man came back, the hour hand was y divisions ahead of 5'O clock.

Since the minute-hand and hour-hand exactly interchanged places during the interval that the man remained out, it is clear that when the man went out, the minute-hand was at y and hour-hand was at x, and when the man came back the minute-hand was at x and the hour-hand was at y.

We know that the speed of the hour hand and minute hand are in the ratio 1 : 12.
From the above diagram, In the time hour hand moves x divisions, hour hand moves 25 + y divisions.  (calculate from 4'O clock)
⇒112=x25+y$\Rightarrow {\displaystyle \frac{1}{12}={\displaystyle \frac{x}{25+y}}}$ ----------- (1)
Also in the time hour hand moves y divisions, minute hand moves 10 + x divisions (calculate from 5'O clock)
⇒112=y20+x$\Rightarrow {\displaystyle \frac{1}{12}={\displaystyle \frac{y}{20+x}}}$ ----------- (2)

From equation (1) we get 25 + y = 12x and from equation (2) we get 20 + x = 12y or x = 12 y - 20
Substituting x value in equation (1)
⇒$\Rightarrow$25 + y = 12 (12y - 20)
⇒$\Rightarrow$25 + y = 144y - 240
⇒$\Rightarrow$143 y = 265
⇒$\Rightarrow$ y = 1122143$1{\displaystyle \frac{122}{143}}$

So the person went out y divisions after 5.  So 25 + 1122143$1{\displaystyle \frac{122}{143}}$

So the time he went out = 4 hours 26122143$26{\displaystyle \frac{122}{143}}$

Alternate method:

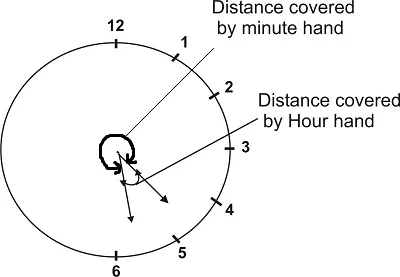
From the above diagram it is clear that Hour hand and Minute hand together covered 60 minute spaces.
We know that the speeds of hour hand and minute hand are in the ratio 1 : 12.  So our of these 60 minute spaces hour hand would have covered 60×113=4813$60\times {\displaystyle \frac{1}{13}=4{\displaystyle \frac{8}{13}}}$ minute spaces.

i.e., Minute hand is 4813$4{\displaystyle \frac{8}{13}}$ minute spaces ahead of hour hand when the man went out.

At 4'O clock Minute hand is 20 minute spaces behind hour hand..  When the man went out it was 4813$4{\displaystyle \frac{8}{13}}$ spaces ahead of hour hand.  So it has gained 20 + 4813$4{\displaystyle \frac{8}{13}}$ = 20 + 6013${\displaystyle \frac{60}{13}}$ = 32013${\displaystyle \frac{320}{13}}$

But we know that minute hand gains 55 minute spaces over the hour hand in 60 minutes.
It gains 1 minute space in 6055${\displaystyle \frac{60}{55}}$ minutes.
To gain 32013${\displaystyle \frac{320}{13}}$ minute spaces it takes 32013×6055=26122143${\displaystyle \frac{320}{13}\times {\displaystyle \frac{60}{55}=26{\displaystyle \frac{122}{143}}}}$ minutes.

So the man went out at 4 hours 26122143$26{\displaystyle \frac{122}{143}}$ minutes.

8. A person who left home between 2 p.m. and 3 p.m. returned between 4 p.m. and 5 p.m. and found that the hands of his watch had exactly changed places. How much time did he out?

In this questions, Hour hand and minute hand together covered 120 minute spaces together.  (2:mm to 4:mm)
Of these minute hand would have covered 12/13 part.
So total time he went out given by ⇒120×1213=1101013$\Rightarrow 120\times {\displaystyle \frac{12}{13}=110\frac{10}{13}}$ minutes.

9. Between 5 and 6 a lady looked at her watch and mistaking hour hand for the minute hand, she thought that she was 57 minutes earlier than the correct time.  When was the correct time?

Let hour hand is x minute spaces ahead of 5. As we know hour hand speed is 12 times of hour hand, Minute hand moved 12x minute spaces.
So correct time = 5: 12x or (300 + 12 x) minutes
But she mistook this time and assumed 4 : (25 + x) or (265 + x) minutes
⇒$\Rightarrow$ (300 + 12x) - (265 + x) = 57 minutes
⇒$\Rightarrow$ x = 12 min

So correct time = 5: 24 minutes.


10. When asked about the time, Amit replied; "If you add one quarter of the time from midnight till now to half the time from now till the next midnight, you get the time".  what is the time now?
Let the time be "t" hours.  From mid night till this time "t" hours passed.  From now to next midnight there are (24-t) hours.

Now
t4+24−t2=t⇒t+48−2t4=t${\displaystyle \frac{t}{4}+\frac{24-t}{2}=t\Rightarrow \frac{t+48-2t}{4}=t}$
⇒48−t=4t$\Rightarrow 48-t=4t$
⇒5t=48⇒t=485$\Rightarrow 5t=48\Rightarrow t={\displaystyle \frac{48}{5}}$hours or 935$9{\displaystyle \frac{3}{5}}$ hours

11. The inhabitants of planet Rahu measure time in hours and minutes which are different from the hours and minutes of our earth.  Their day consists of 36 hours with each hour having 120 minutes.  The dials of their clocks show 36 hours.  What is the angle between the hour hand and the minutes hand of a Rahuian clock when it shows a time of 9:48? [Rahuians measure angles in degrees the way we do on earth.  But for them, the angle around a point is 720 degrees instead of 360 degrees. ]
In rahuian degrees the minutes hand travels a full circle in 1 hour.  i.e., 120 minutes
i.e., 720 degrees in 120 min or 6 degrees per min
and the hours hand travels 720/36= 20 degrees per hour
and 20/120 = 1/6 degrees/minute.
At 9:48 the hours:
Angle covered by hour hand = 9 x 20 + 48 x 1/6 = 188 deg
Angle covered by min hand = 48 x 6 = 288 deg
Angle between hour hand and minute hands = 288 - 188 = 100 degrees

FacebookTwitterGoogle+Share